
Students communicate clearly and express themselves creatively for a variety of purposes using the platforms, tools, styles, formats and digital media appropriate to their goals. Make connections between visual arts and other disciplines. Understand the visual arts in relation to history and cultures. Consortium of National Arts Education Associations Understand that a two-dimensional figure is similar to another if the second can be obtained from the first by a sequence of rotations, reflections, translations, and dilations given two similar two-dimensional figures, describe a sequence that exhibits the similarity between them. Introduction to Tessellations ISBN: 0866514619 Standards Common Core Standards for Math:Ĩ.G.A.4. Escher: Visions of Symmetry ISBN: 0810943085
#Easy tessellation pattern for free
You may want to put the final images into your students’ portfolios and have them record their reflections on the process.Ĭreate your own rubric for free at Resources As students build their tessellations, you can evaluate their mathematical understanding of symmetry as well as visual skill using layers and colors. AssessmentĪs you activate prior knowledge by describing floor and ceiling tiles and examining the images created by Escher, you will have an idea of how well your students understand symmetry and how shapes can be tessellated. In this case, a student-created introduction to tessellations and M.C. You could also post the student work to your Web site. You might even want to have them create printed handouts to share with gallery visitors. Have your students act as docents to explain more about tessellations and the works of Escher. Invite parents, school leaders, and other classes to come in and view the images in your gallery. Have students print their tessellations and turn your room into an Escher-esque gallery. It is easy to create reflection, rotation, and translation tessellations in tools like Pixie or Wixie. The computer makes this exercise so much easier! You can create tessellations on paper, but it can be time consuming and tedious. Students should look closely at the art and discuss the original shapes Escher used to create the art. Once the class has watched the interviews, review his symmetry prints in the picture gallery. Explore the four types of symmetry in a plane – reflection, translation, rotation, and glide rotation – and how these can be used to create tessellations.Īs a class, watch the interviews with Escher on the official M.C. Triangles, rectangles, and parallelograms also tessellate.ĭraw each shape on the board and invite students to draw lines of symmetry on each shape and explain how symmetry allows the shapes to tessellate. Squares are easy, and, in fact, the word tessellate comes from the Greek word “tesseres,” which means “four.” A simple tessellation is an arrangement of small squares in a checkered or mosaic pattern. Work together to brainstorm shapes that tessellate.
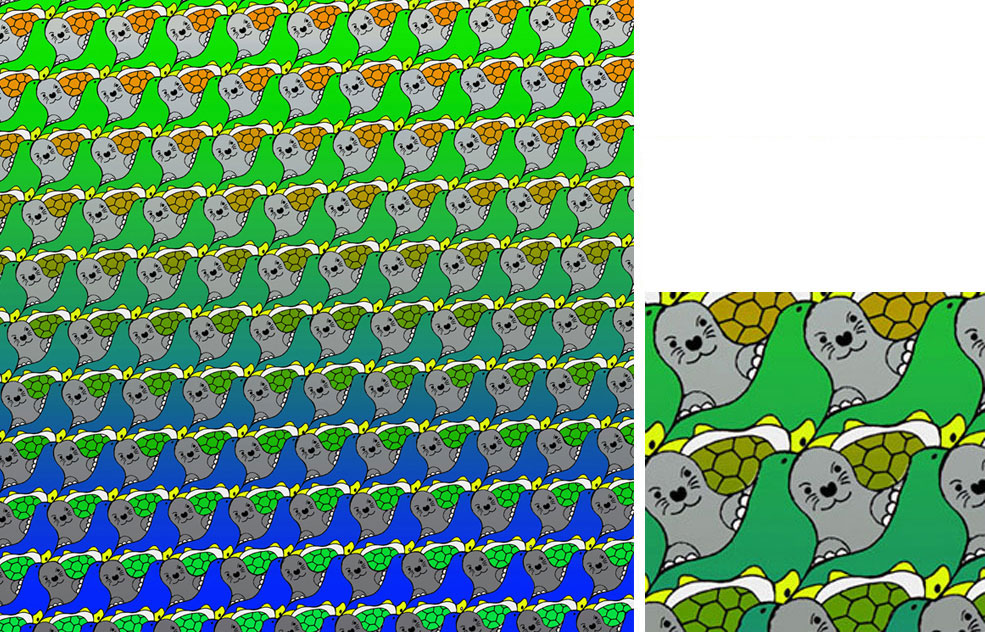
What does the artwork have in common with the floor or ceiling tiles in the classroom? Ask your students if they recognize the work of art and can name the artist.

You might consider using Development I, 1937 (rotation), Day and Night, 1938 (reflection), or Sky and Water I, 1938 (translation). Share a few examples of tessellations create by Escher. You should hear words like patterns, shapes, squares, symmetry, mosaic, and planes. Exploring tessellations and the art of Escher is a great way to help students make these connections.Īs students enter your classroom, ask them to look at the floor or ceiling tiles and use math terms to describe them. The connections between art and math are evident and numerous, yet often overlooked. Your work will be displayed in your school’s own Escher-esque gallery. In this activity, you will learn the mathematical concepts of pattern and symmetry and then create your own Escher-like art in the form of tessellations. Escher was an illustrator known for his very precise, scientific, and mathematical drawings. Many of the concepts you learn in a math classroom can be found in other school subjects… including art. Escher in order to create their own tessellations.

Students will study the mathematical concepts of patterns, planes, symmetry, and translation and learn about the work of M.C.


 0 kommentar(er)
0 kommentar(er)
